a. D
45. Both equations of the law of ABSORPTION, A(A+B) =A and
b. AQ
A+(AB) =A can be proved by applying the laws of Boolean Algebra
c. X
shown below.
d. EZ
A(A+B) =A
AA+AB=A
DISTRIBUTIVE
A+AB=A
IDEMPOTENT
A(1 +B) =A
DISTRIBUTIVE
A 1=A
UNION
A=A
INTERSECTION
A+(AB) =A
A+AB=A
ASSOCIATIVE
A(1 +B) =A
DISTRIBUTIVE
A 1=A
UNION
A=A
INTERSECTION
Since both equations are equal to A, it is mathematically correct
that A(A+B) =A+AB.
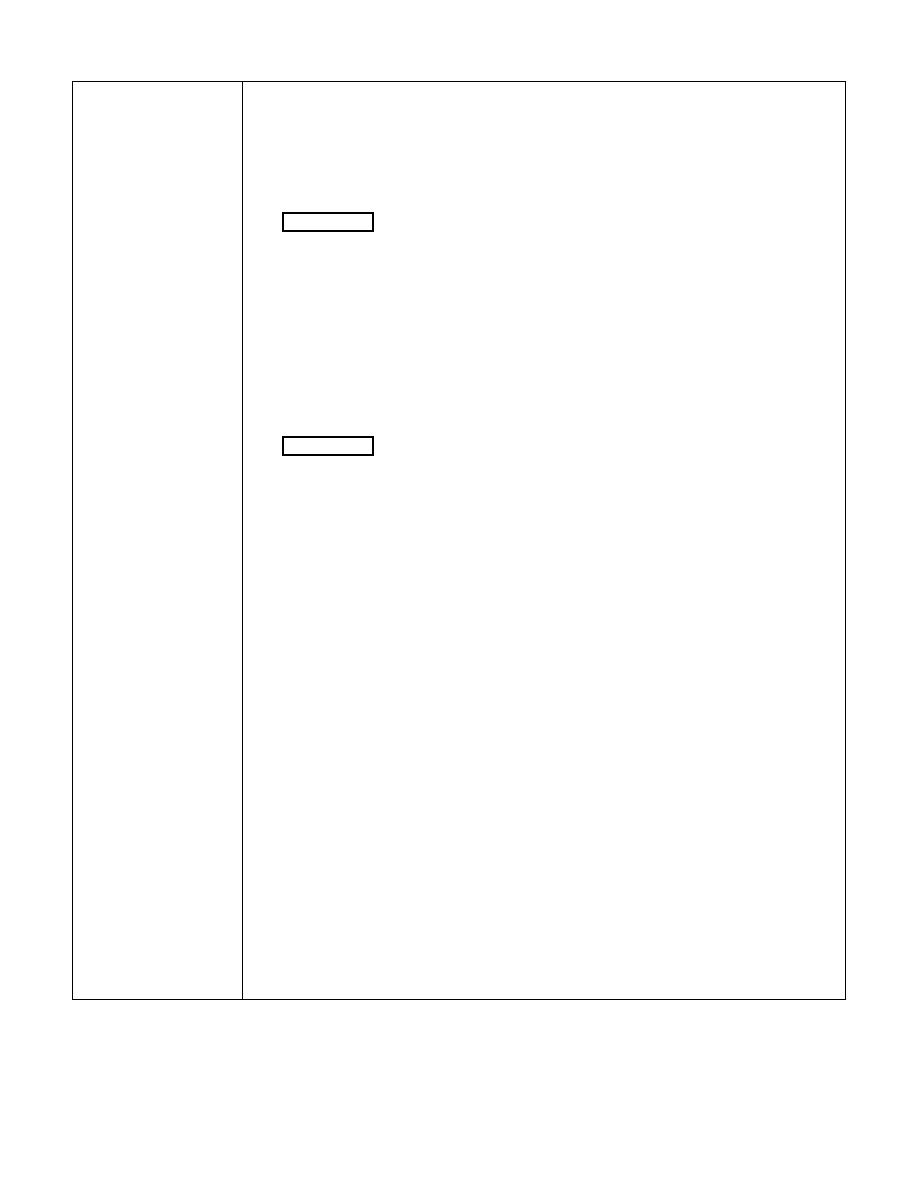
Simplify the following Boolean expressions, using the law of
ABSORTPION.
a. D+DE
b. K+KL+KM
c. TGNE+T+TI
d. V+W+WX
1-31
IT 0344
Previous Page
